-
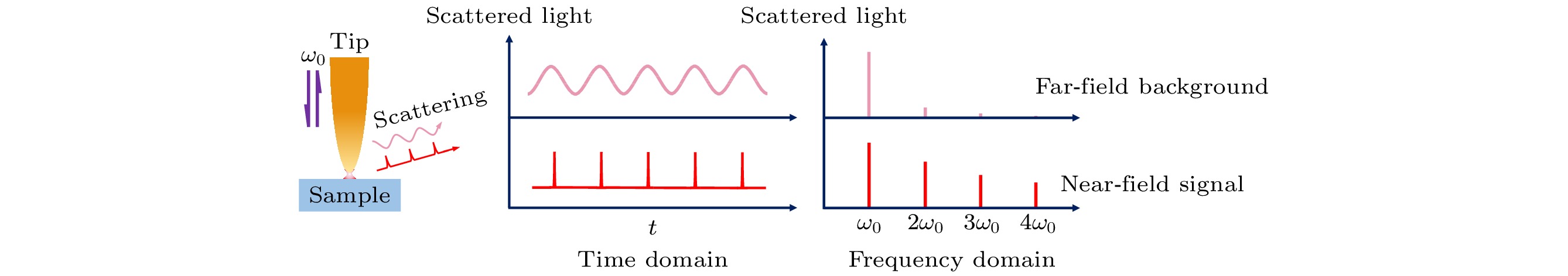
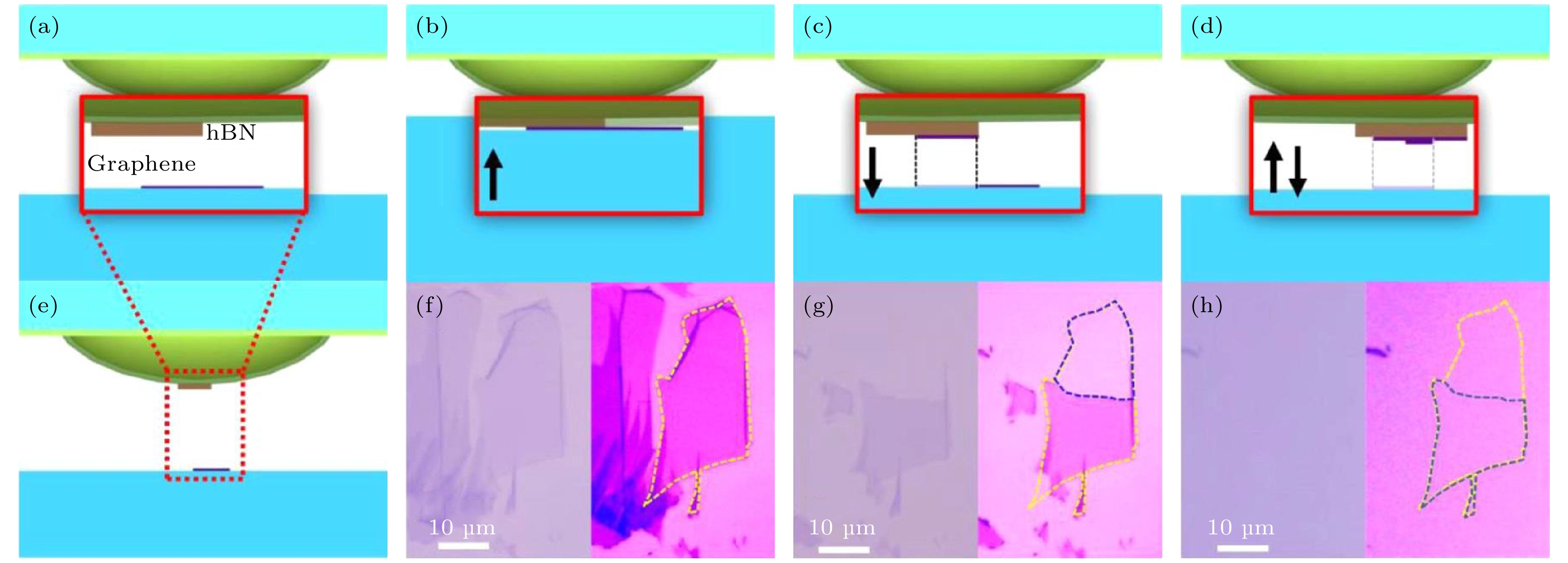
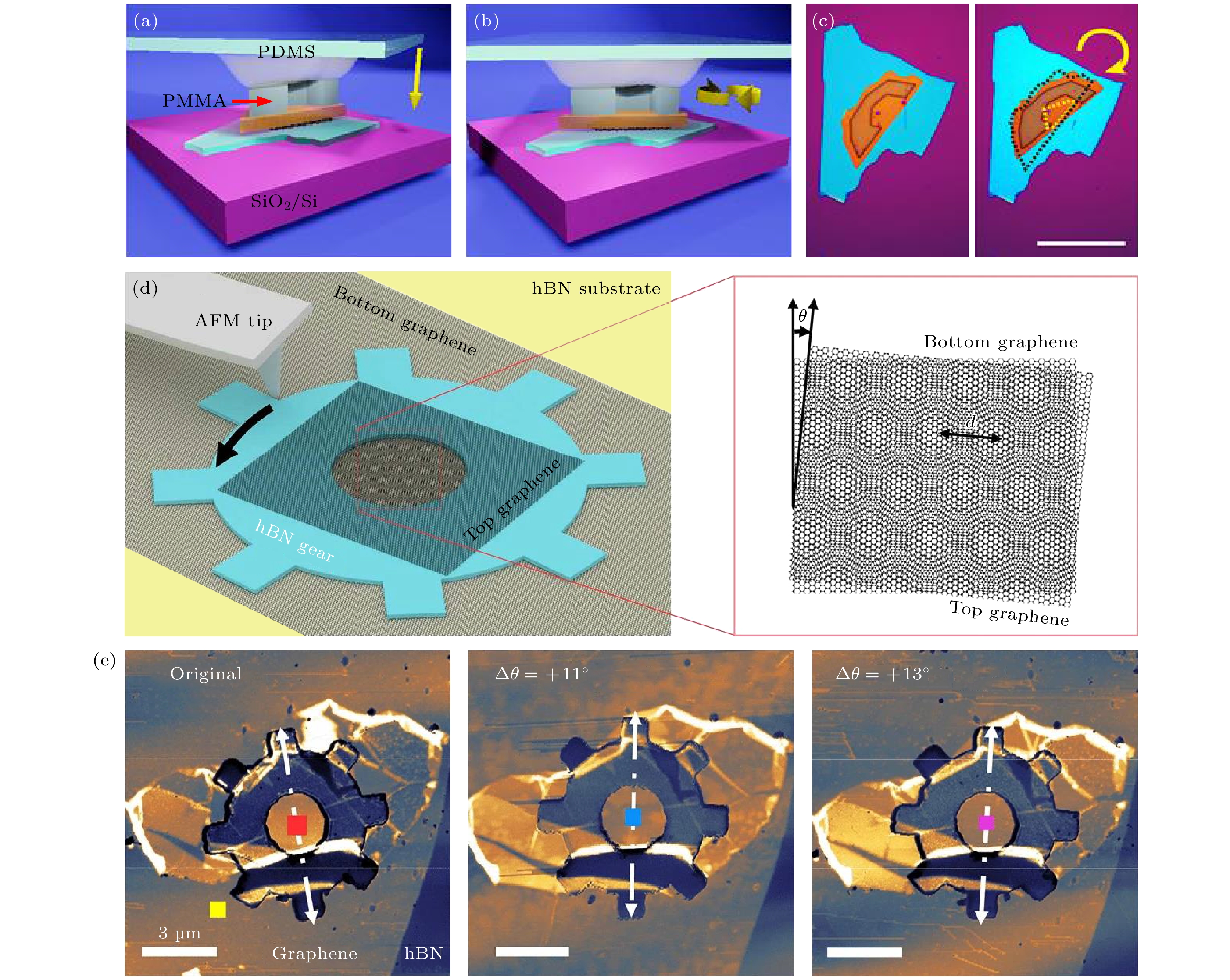
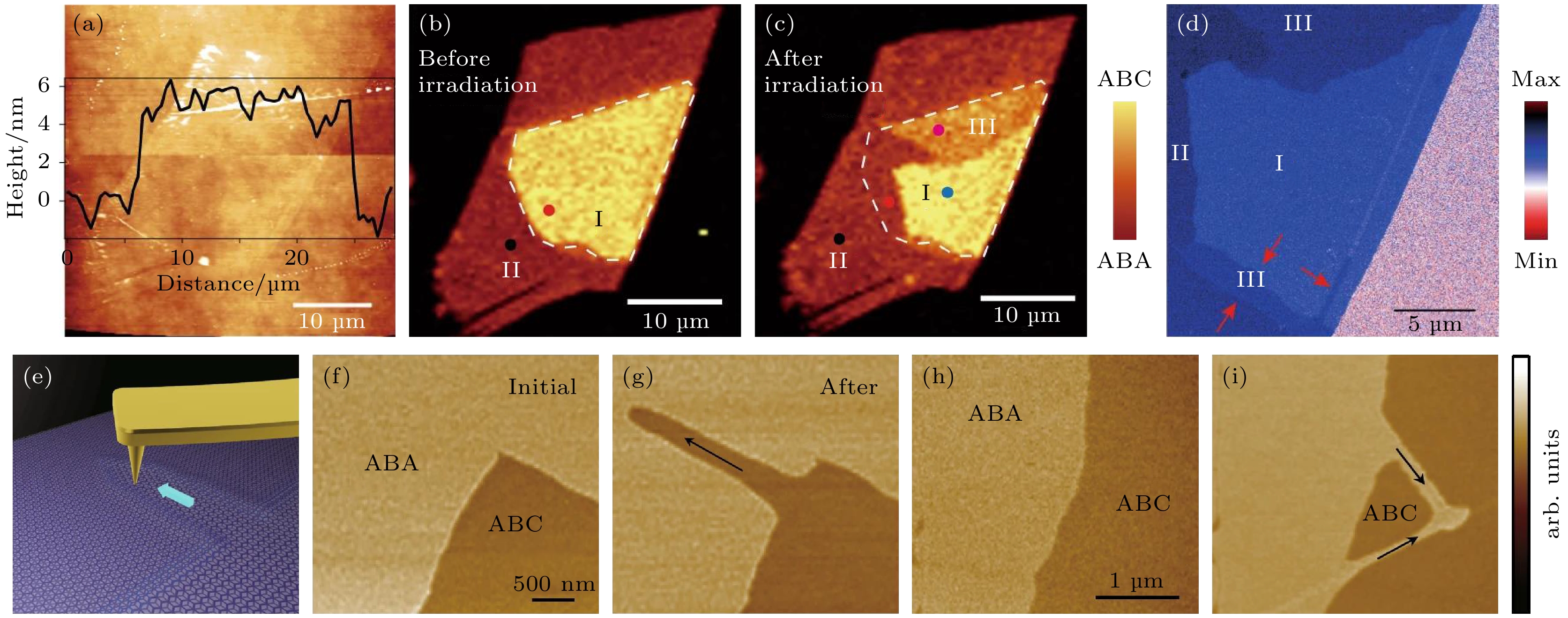
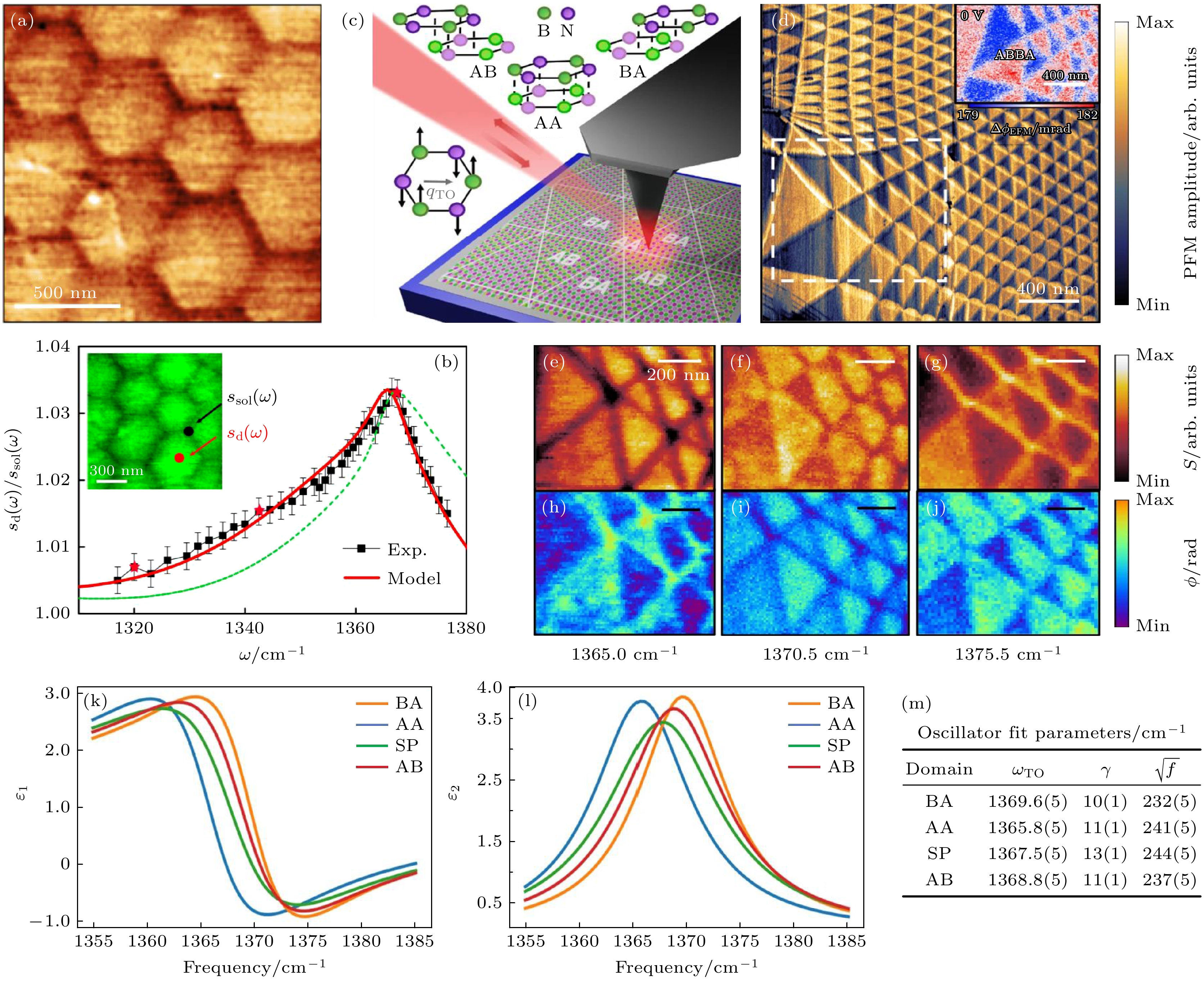
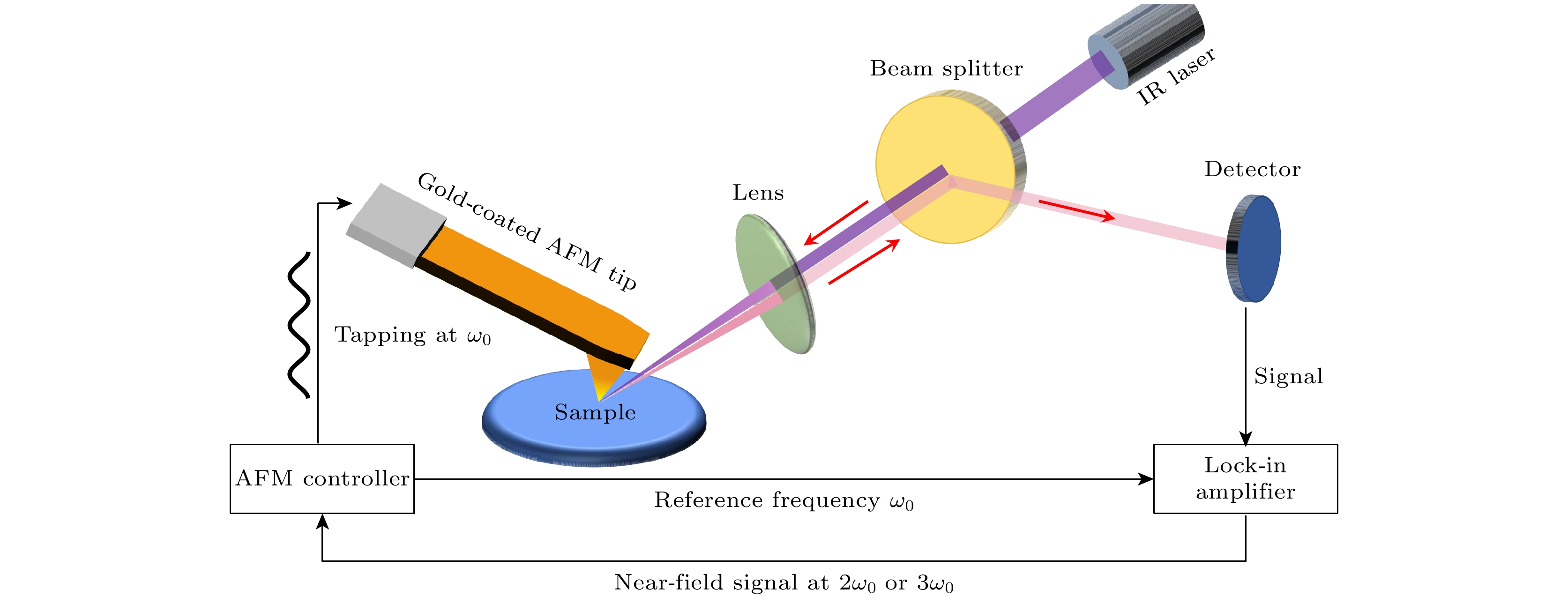
Polariton is a quasiparticle generated from strong interaction between a photon and an electric or magnetic dipole-carrying excitation. These polaritons can confine light into a small space that is beyond the diffraction limit of light, thus have greatly advanced the development of nano photonics, nonlinear optics, quantum optics and other related research. Van der Waals two-dimensional (2D) crystals provide an ideal platform for studying nano-polaritons due to reduced material dimensionality. In particular, stacking and twisting offer additional degree of freedom for manipulating polaritons that are not available in a single-layer material. In this paper, we review the near-field optical characterizations of various structures and polaritonic properties of stacked/twisted 2D crystals reported in recent years, including domain structures of stacked few-layer graphene, moiré superlattice structures of twisted 2D crystals, twisted topological polaritons, and twisted chiral plasmons. We also propose several exciting directions for future study of polaritons in stacked/twisted 2D crystals.
-
Keywords:
- twisted two-dimensional crystals/
- polaritons/
- moiré superlattice/
- scanning near-field optical microscopy
[1] [2] [3] [4] [5] [6] [7] [8] [9] [10] [11] [12] [13] [14] [15] [16] [17] [18] [19] [20] [21] [22] [23] [24] [25] [26] [27] [28] [29] [30] [31] [32] [33] [34] [35] [36] [37] [38] [39] [40] [41] [42] [43] [44] [45] [46] [47] [48] [49] [50] [51] [52] [53] [54] [55] [56] [57] [58] [59] [60] [61] [62] [63] [64] [65] [66] [67] [68] [69] [70] [71] [72] [73] [74] [75] [76] [77] [78] [79] [80] [81] [82] [83] [84] [85] [86] [87] [88] [89] [90] [91] [92] [93] [94] [95] [96] [97] [98] [99] [100] [101] [102] [103] [104] [105] [106] -
[1] [2] [3] [4] [5] [6] [7] [8] [9] [10] [11] [12] [13] [14] [15] [16] [17] [18] [19] [20] [21] [22] [23] [24] [25] [26] [27] [28] [29] [30] [31] [32] [33] [34] [35] [36] [37] [38] [39] [40] [41] [42] [43] [44] [45] [46] [47] [48] [49] [50] [51] [52] [53] [54] [55] [56] [57] [58] [59] [60] [61] [62] [63] [64] [65] [66] [67] [68] [69] [70] [71] [72] [73] [74] [75] [76] [77] [78] [79] [80] [81] [82] [83] [84] [85] [86] [87] [88] [89] [90] [91] [92] [93] [94] [95] [96] [97] [98] [99] [100] [101] [102] [103] [104] [105] [106]
Catalog
Metrics
- Abstract views:5667
- PDF Downloads:413
- Cited By:0













 DownLoad:
DownLoad: