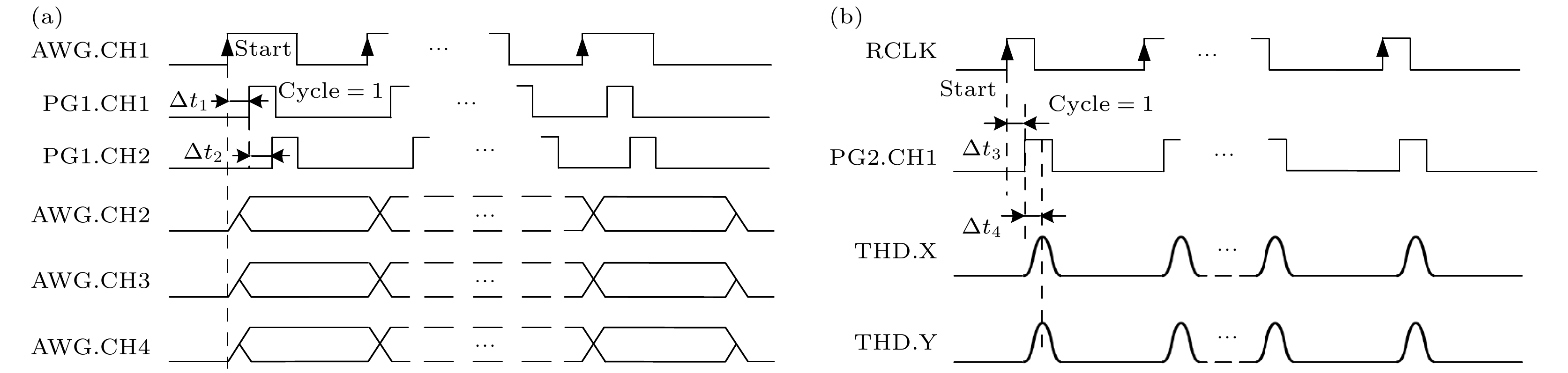
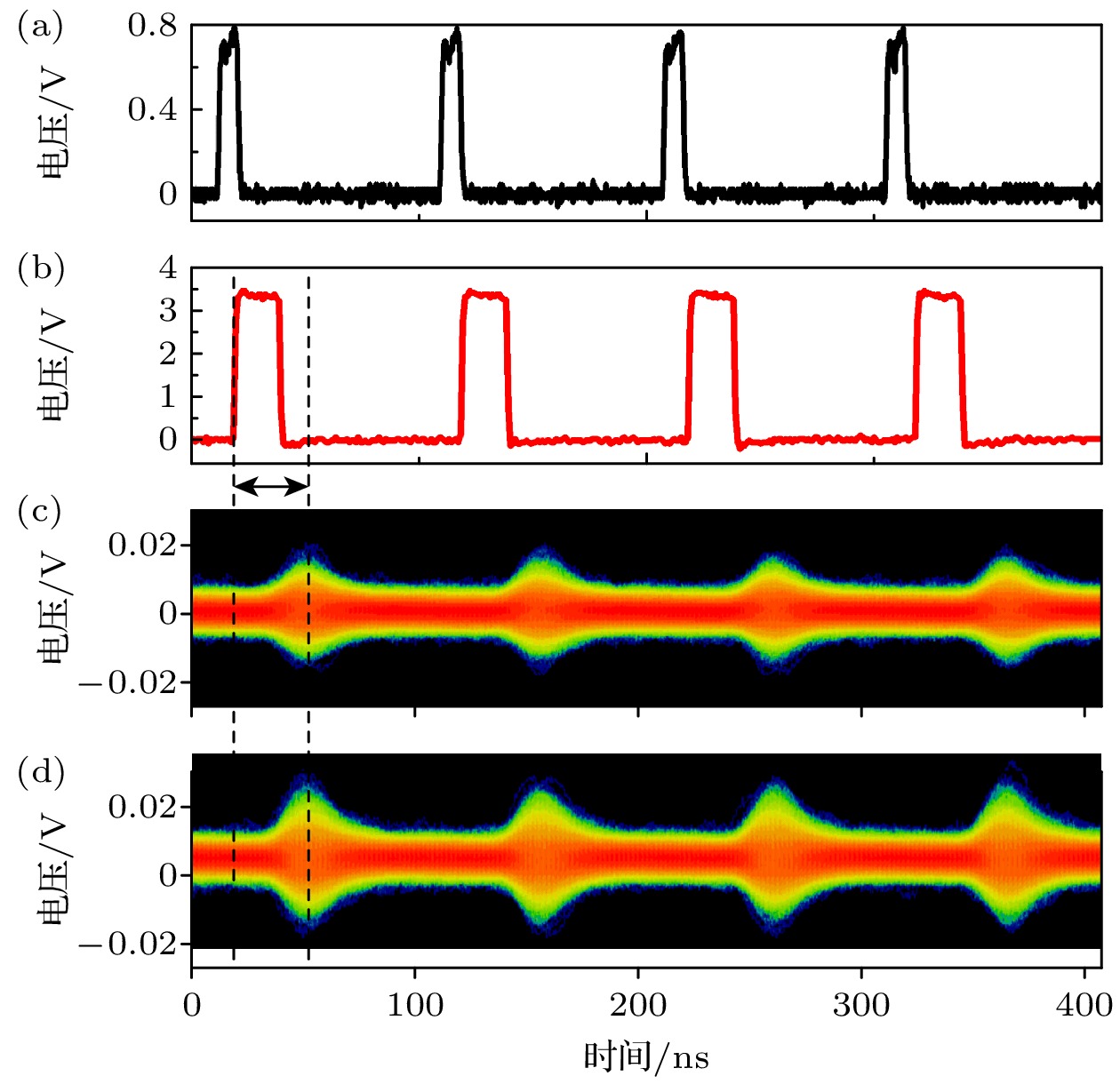
In the case of continuous-variable quantum key distribution (CV-QKD) systems, synchronization is a key technology that ensures that both the transmitter and receiver obtain corresponding data synchronously. By designing an ingenious time sequence for the transmitter and receiver and using the peaking value acquisition technique and time domain heterodyne detection, we experimentally realize a four-state discrete modulation CV-QKD with a repetition rate of 10 MHz, transmitting over a distance of 25 km. With well-designed time sequence of hardware, Alice and Bob can obtain corresponding data automatically without using numerous software calculation methods.
The secure key rates are calculated by using the method proposed by the Lütkenhaus group at the University of Waterloo in Canada. In the calculation, we first estimate the first and the second moment by using the measured quadratures of displaced thermal states, followed by calculating the secret key rate by using the convex optimization method through the reconstruction of the moments. There is no need to assume a linear quantum transmission channel to estimate the excess noise. Finally, secure key rates of 0.0022—0.0091 bit/pulse are achieved, and the excess noise is between 0.016 and 0.103.
In this study, first, we introduce the prepare-and-measure scheme and the entanglement-based scheme of the four-state discrete modulation protocol. The Wigner images of the four coherent states on Alice’s side, and four displaced thermal states on Bob’s side are presented. Second, the design of hardware synchronization time series is introduced comprehensively. Third, the CV-QKD experiment setup is introduced and the time sequence is verified. Finally, the calculation method of secure key rate using the first and the second moment of quadrature is explained in detail. The phase space distribution of quadratures is also presented. The secret key rate ranges between 0.0022 and 0.0091 bits/pulse, and the equivalent excess noise are between 0.016 and 0.103. The average secret key bit rate is 24 kbit/s. During the experiment, the first and the second moment of the quantum state at the receiver end are found to fluctuate owing to the finite-size effect. This effect reduces the value of the secure key rate and limits the transmission distance of the CV-QKD system.
In conclusion, four-state discrete modulation CV-QKD based on hardware synchronization is designed and demonstrated. The proposed hardware synchronization method can effectively reduce the cost, size, and power consumption. In the future, the finite-size effect will be investigated theoretically and experimentally to improve the performance of system.














 下载:
下载: