-
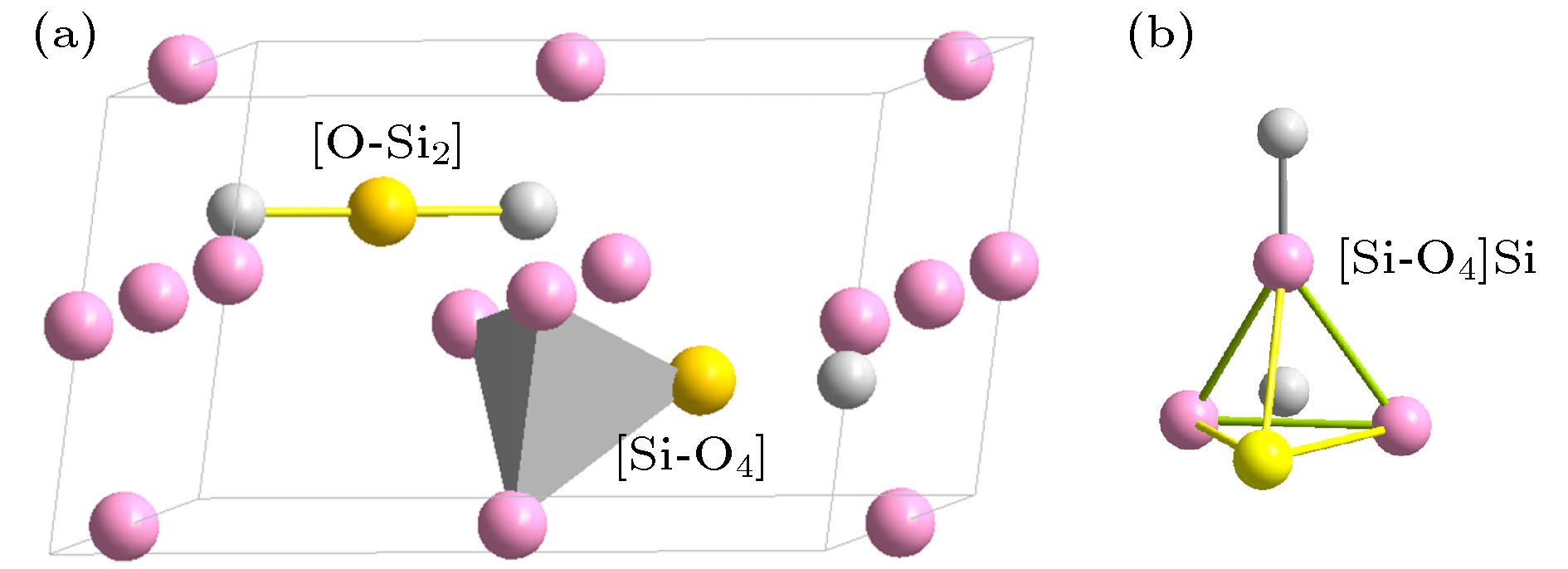
本文引入团簇加连接原子模型来解析硅酸盐玻璃相关氧化物的结构, 并给出了这些氧化物的最小结构单元——类分子结构单元. 参与网络形成体的氧化物主要以三角形或者四面体的配位体形式存在, 构建玻璃的三维网络状骨架. 如基础的网络形成体SiO 2, 其类分子结构单元团簇式是为[Si-O 4]Si, 含有的价电子数为32, 形成四面体网络. 中间体以同时形成八面体和四面体为特征, 网络外体则以立方体和八面体为主. 经证实, 这些类分子结构单元无一例外, 均满足八电子规则(即每个结构单元所含的价电子总数为8的整数倍), 具有分子的属性. 玻璃中氧化物类分子结构单元概念的提出, 将为后续的玻璃成分设计工作打下基础.Silica glasses are composed of multi-oxides, apart from the major component silica. Though it is a general practice in the industries to prepare glasses at specific oxide ratios, the composition rule is largely missing, complicated by the implication of multi-oxides. Necessarily, their interpretation is rooted in chemical units, on which the specific compositions depend. However, in silica glasses the inter-atomic bonding network is continuous and there is no weak bonds, like the inter-molecular ones in molecular compounds, to define molecular entities that carry the chemical information of the materials. As the first stage towards understanding the composition rule, the present paper introduces a new method, so-called the cluster-plus-glue-atom model, to unveil the molecule-like structural units of the glass-relevant oxides. It is pointed out that their respective contributions to the construction of glass networks originate from their characteristic cluster structures, and from which molecule-like structural units are proposed that represent the smallest structural units of these oxides. Oxides participating in the glass network formation mainly present triangular or tetrahedral clusters which are required for a three-dimensional glassy network. For example, the basic network former SiO 2is formulated as [Si-O 4]Si and contains 32 valence electrons. The intermediate oxides are characterized by the simultaneous formation of both octahedra and tetrahedra. The network modifiers present mainly cubes and octahedra. It is confirmed that the molecule-like structural units of the glass-formation oxides all meet octet rule (that is, the total number of valence electrons contained in each structural unit is an integer multiple of 8), just like common molecules. The proposed concept of molecular structural units sheds a new light on understanding the composition rule of silicate glasses and can eventually solve the long-standing problem of composition design of silica glasses.
[1] [2] [3] [4] [5] [6] [7] [8] [9] [10] [11] [12] [13] [14] [15] [16] [17] [18] [19] [20] [21] [22] [23] [24] [25] [26] -
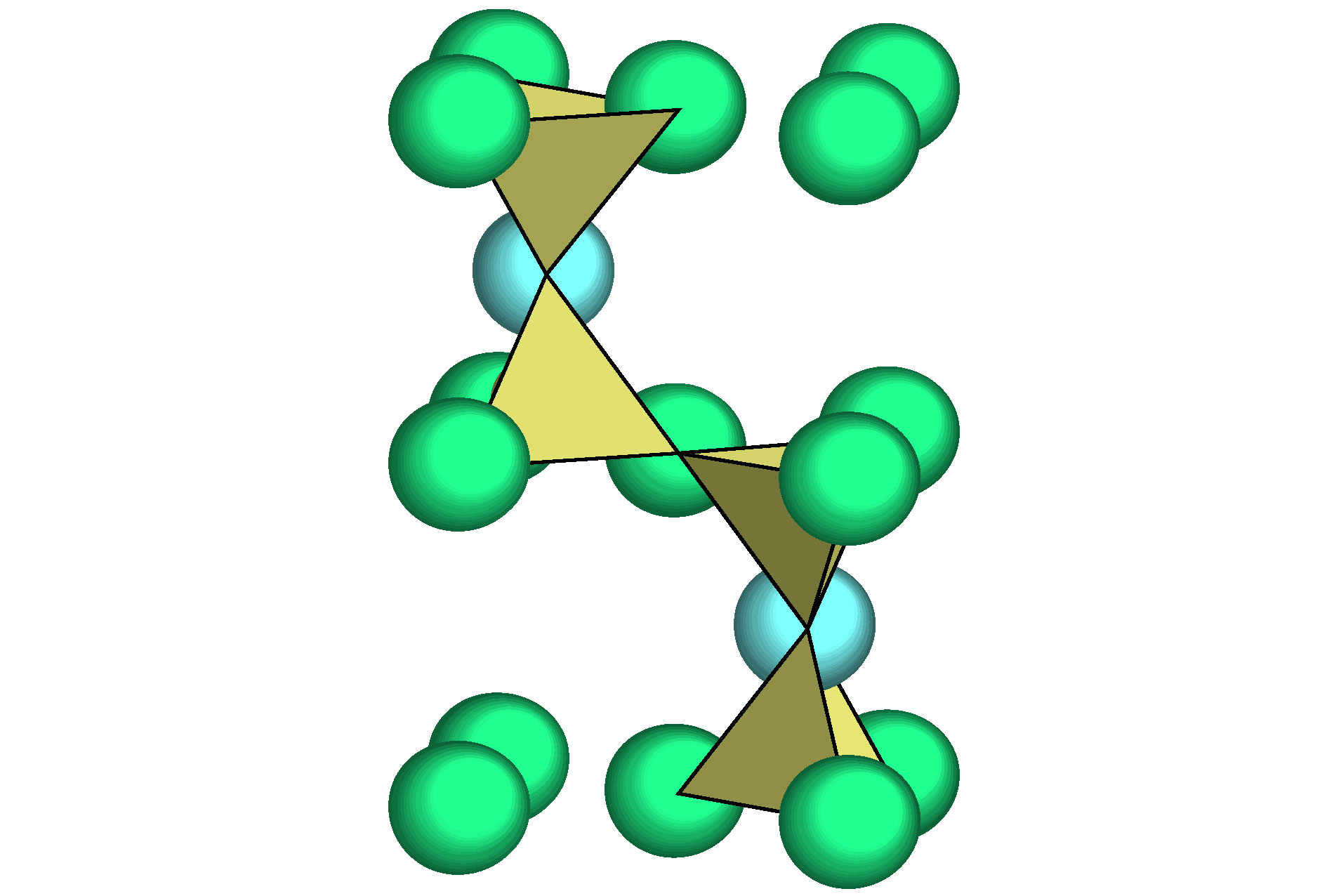
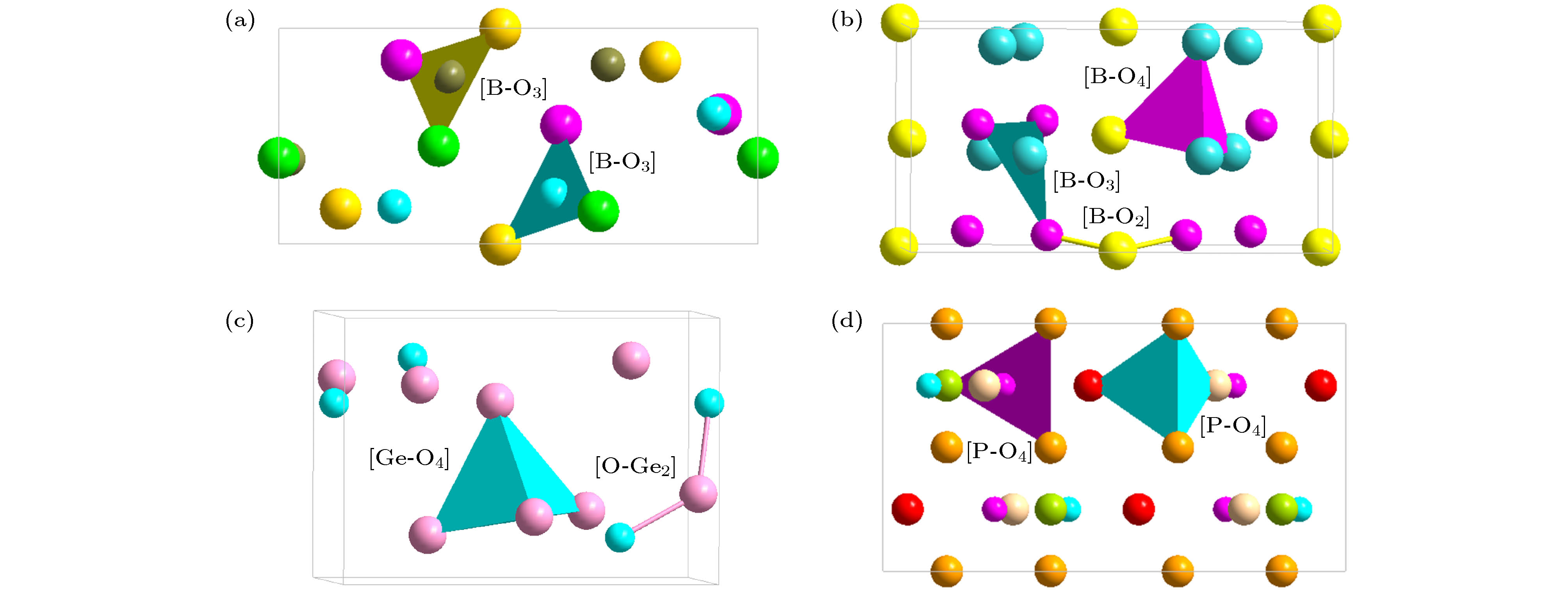
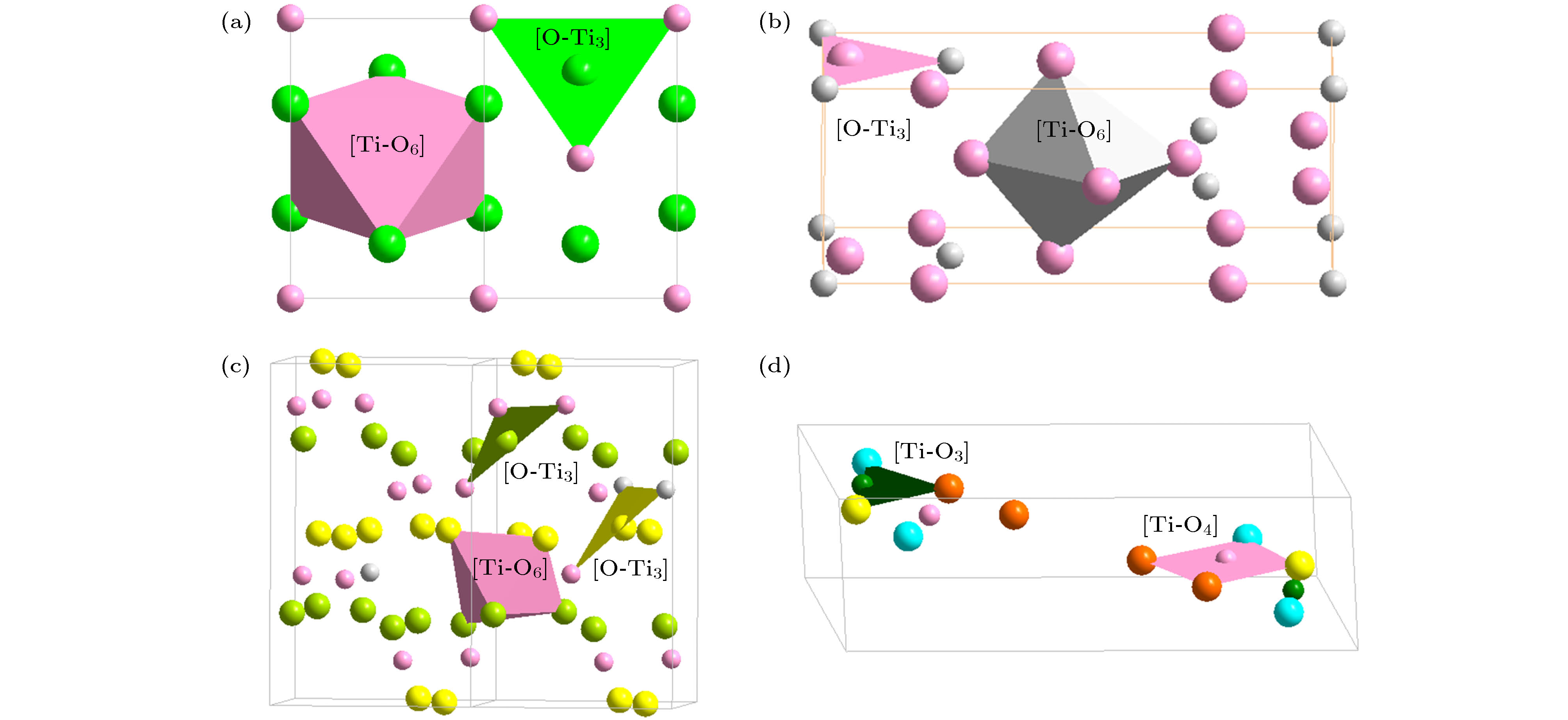
O2Si Structure type O2Si Pearson symbolhP12 Space groupP63/mmc No.194 a= 0.5052(9) nm c= 0.827(2) nm γ= 120° O1 2c $ {\bar 6}m2$ x= 1/3 y= 2/3 z= 1/4 occ. = 1 Si 4f 3m. x= 1/3 y= 2/3 z= 0.0620 occ. = 1 O2 6g .2/m x= 1/2 y= 0 z= 0 occ. = 1 Classification Oxide (space group) Cationic structural unit (e/u) Anion structural unit (e/u) Network formation β-SiO2(P63/mmc) [Si-O4]Si= 2{SiO2} (32) [O-Si2]O3= 2{SiO2} (32) B2O3(P31) [B-O3]B= {B2O3} (24) [O-B2]O2= {B2O3} (24) B2O3(Cmc21) [B-O4]B3O2= 2{B2O3} (48) [O-B2]O2= {B2O3} (24) [O-B3]O5B1= 2{B2O3} (48) GeO2(P41212) [Ge-O4]Ge= 2{GeO2} (32) [O-Ge2]O3= 2{GeO2} (32) P2O5(Pnma) [P-O4]P1O1= {P2O5} (40) [O-P2]O4= {P2O5} (40) [O-P1]P1O4= {P2O5} (40) Network outside body (Li, Na,K)2O
(anti-fluorite$ Fm{\bar 3}m$)[(Li, Na,K)-O4](Li, Na,K)7=
4{(Li, Na,K)O2} (32)[O-(Li, Na,K)8]O3=
4{(Li, Na,K)O2} (32)(Mg, Ca, Ba)O
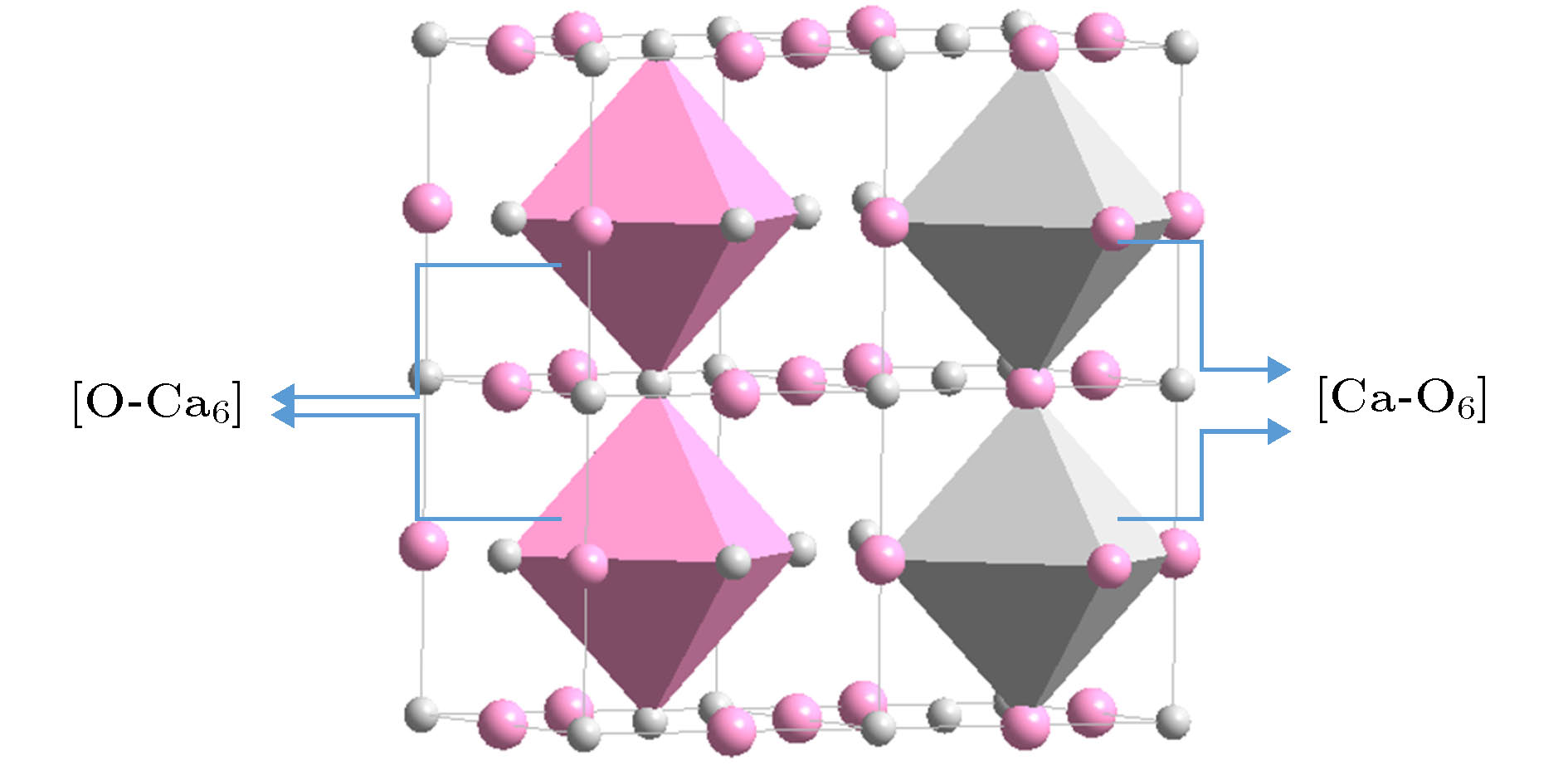
(Halite, $ Fm{\bar 3}m$)[(Mg, Ca, Ba)-O6](Mg, Ca, Ba)5=
6{(Mg, Ca, Ba)O} (48)[O-(Mg, Ca, Ba)6]O5=
4{(Mg, Ca, Ba)O} (48)ZrO2(rutile,P42/mnm) [Zr-O6]Zr2= 3{ZrO2} (48) [O-Zr3]O5= 3{ZrO2} (48) Network intermediate (Be, Zn)O
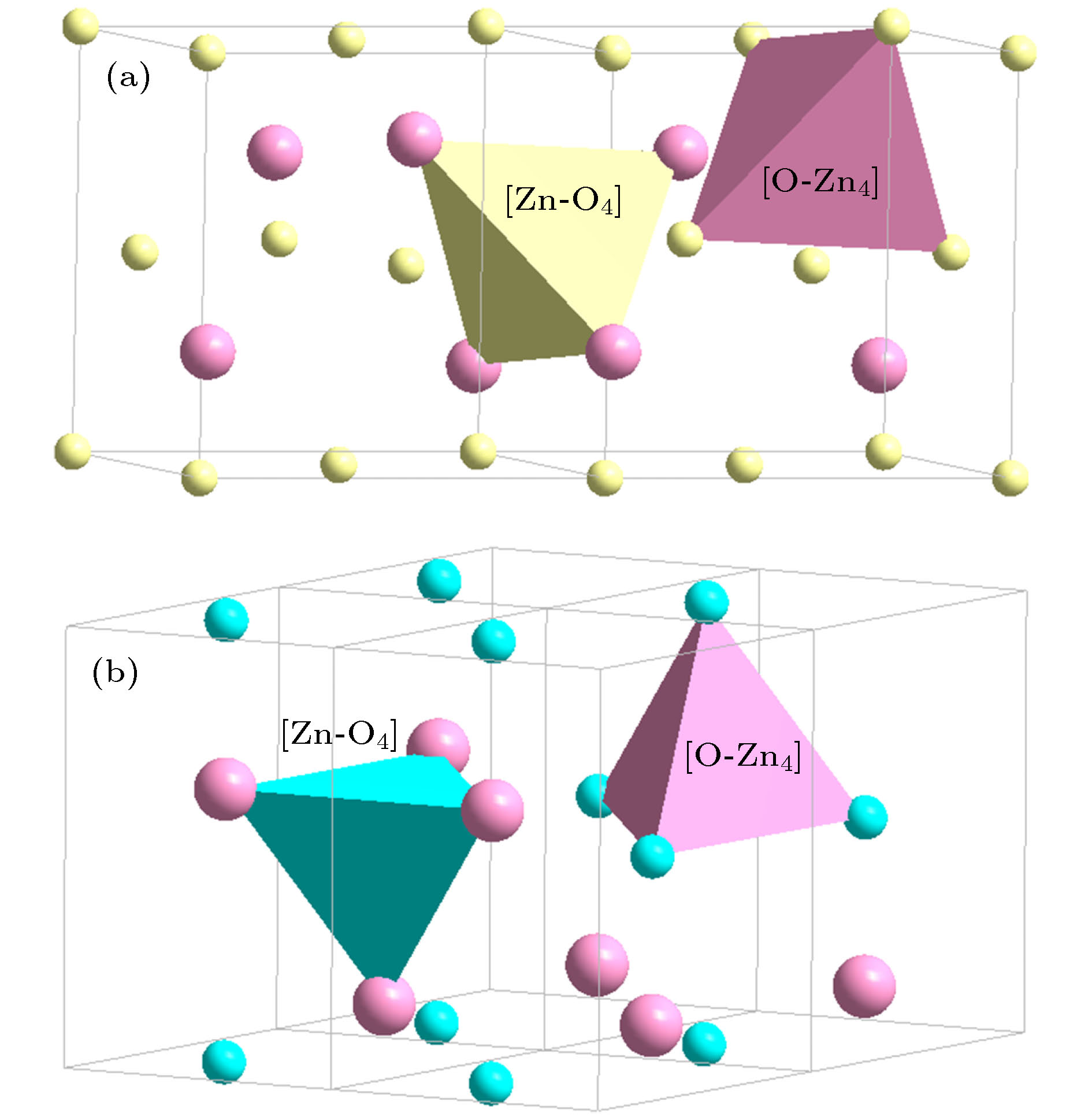
(sphalerite,P43m)[(Be, Zn)-O4](Be, Zn)3= 4{(Be, Zn)O} (32) [O-(Be, Zn)4]O3= 4{(Be, Zn)O} (32) (Be, Zn)O
(Wurtzite,P63mc)[Zn-O4]Zn3= 4{ZnO} (32) [O-Zn4]O3= 4{ZnO} (32) (Be, Zn)O
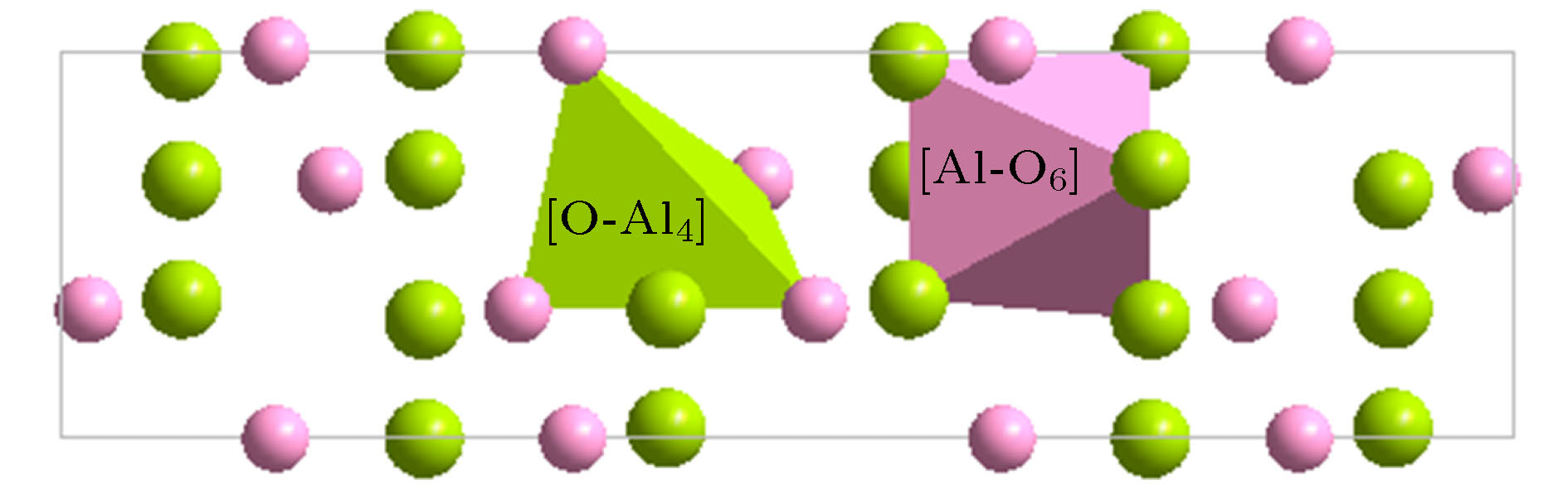
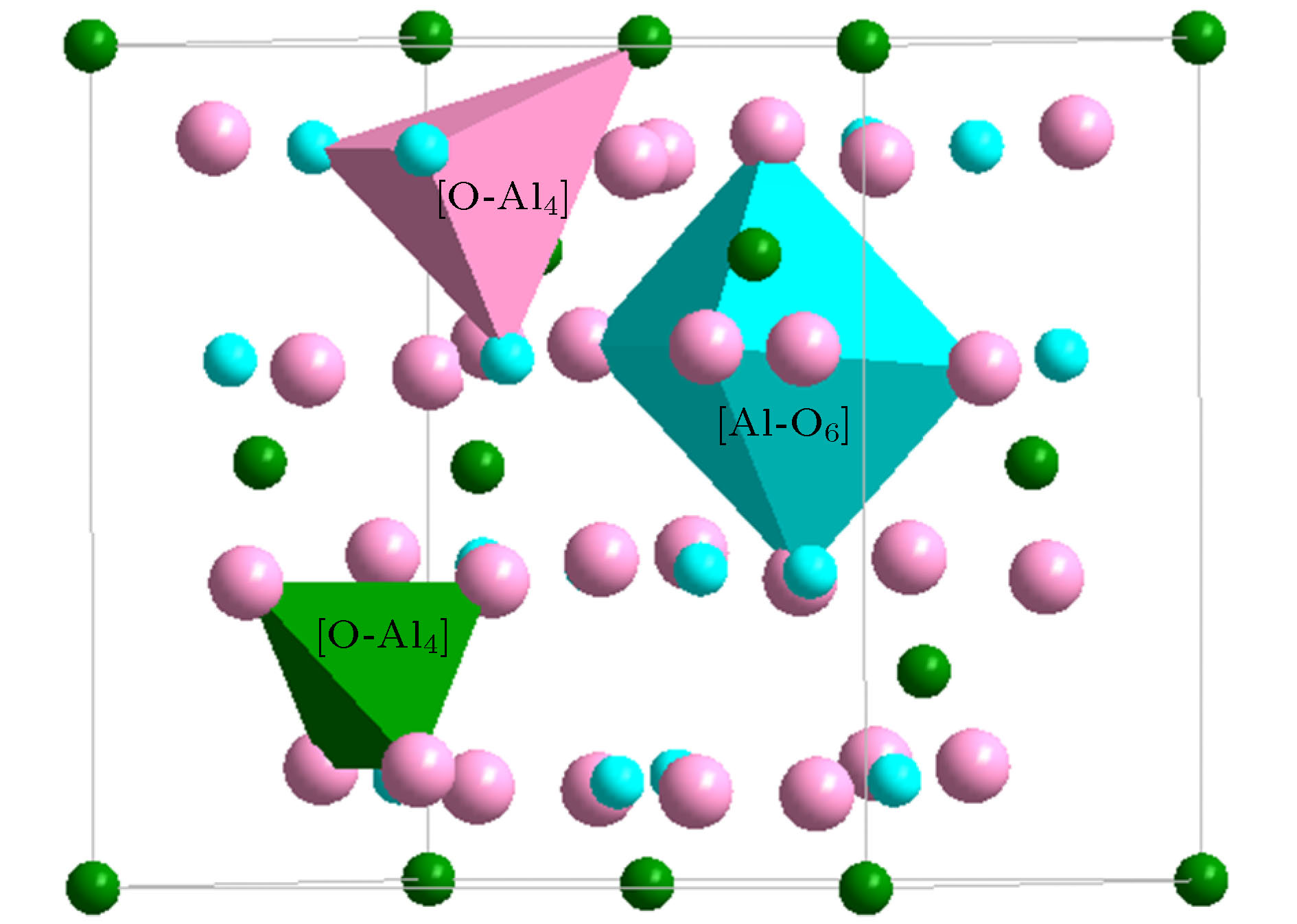
(Halite, $ Fm{\bar 3}m$)[Zn-O6]Zn5= 6{ZnO} (48) [O-Zn6]O5= 6{ZnO} (48) α-Al2O3($ R{\bar 3}c$) [Al-O6]Al3= 2{Al2O3} (48) [O-Al4]O5= 2{Al2O3} (48) γ-Al2O3(Spinel, $ Fd{\bar 3}m$) [Al-O4]O2Al3= 2{Al2O3} (48) [O-Al4]O5= 2{Al2O3} (48) [Al-O6]Al3= 2{Al2O3} (48) Ga2O3(α-Al2O3, $ R{\bar 3}c$) [Ga-O6]Ga3= 2{Ga2O3} (48) [O-Ga4]O5= 2{Ga2O3} (48) Ga2O3(Spinel, $ Fd{\bar 3}m$) [Ga-O4]O2Ga3= 2{Ga2O3} (48) [O-Ga4]O5= 2{Ga2O3} (48) [Ga-O6]Ga3= 2{Ga2O3} (48) Fe2O3(α-Al2O3, $ R{\bar 3}c$) [Fe-O6]Fe3= 2{Fe2O3} (48) [O-Fe4]O5= 2{Fe2O3} (48) Fe3O4(Spinel, $ Fd{\bar 3}m$) [Fe-O4]Fe2= {Fe3O4} (32) [O-Fe4]O7Fe2= 2{Fe3O4} (64) [Fe-O6]Fe5O2= 2{Fe3O4} (64) TiO2(Rutile,P42/mnm) [Ti-O6]Ti2= 3{TiO2} (48) [O-Ti3]O5= 3{TiO2} (48) TiO2(Anatase,I41/amd) [Ti-O6]Ti2= 3{TiO2} (48) [O-Ti3]O5= 3{TiO2} (48) TiO2(Brookite,Pbca) [Ti-O6]Ti2= 3{TiO2} (48) [O-Ti3]O5= 3{TiO2} (48) TiO2(P21/m) [Ti-O4]Ti= 2{TiO2} (32) [O-Ti2]O3= 2{TiO2} (32) [Ti-O3]TiO= 2{TiO2} (32) [O-Ti]O3Ti = 2{TiO2} (32) -
[1] [2] [3] [4] [5] [6] [7] [8] [9] [10] [11] [12] [13] [14] [15] [16] [17] [18] [19] [20] [21] [22] [23] [24] [25] [26]
计量
- 文章访问数:14182
- PDF下载量:172
- 被引次数:0













 下载:
下载: